1. Introduction
The Significant figures make our calculations and estimations more precise and accurate. To increase the understanding of a long number just a round of measurement to make it more precise.
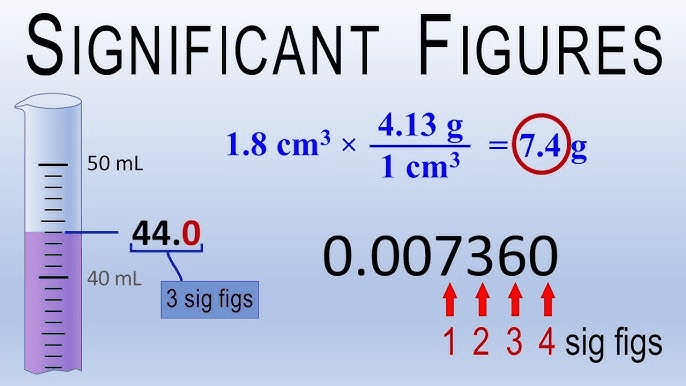
The significant figure calculator rounds off the figure according to our requirements. The significant fingers are necessary for making too-large or too-small measurements more reasonable. Significant figures are used when you are dealing with very small and large quantities in Mathematics.
2. Reason for Rounding A Number
The significant figures are critical when You are going to find precise and accurate results. The significant figures make the estimation more precise and easy to understand. Significant figures are used when you are dealing with very small and large quantities in calculations.
There are certain rules to follow when you are extracting a significant figure from a figure. You do need to implement the significant figure at the workplace to make our estimation more precise and accurate.
Example:
For example, measurements like 5.48966666 cm are not simple to understand but 5.5 cm is simple. The calculator makes the task of rounding simple and fast. You can observe that 5.5 cm is considerably simple to understand compared to 5.48966666 cm. This is the main reason numbers like 5.48966666 cm are rounded to 1 Decimal Place (DP), 2 DP, 3 DP
- 1 DP Rounding Off: 5.48966666 would become 5.5 cm after 4, the number 8 is larger than 5. So add 1 to the first decimal number after the digit. Then 4 becomes 5, and the number would become 5.5 cm.
- 2 DP Rounding Off: 5.48966666 would become 5.49 cm as after 8, the number 9 is larger than 5. So add 1 to the second decimal number after the digit. Then 8 becomes 5, and the number would become 5.49 cm.
- 3 DP Rounding Off: 5.48966666 would become 5.490 cm as after 9, the number 6 is larger than 5. So add 1 to the third decimal number after the digit. Then 9 becomes 10, and the number would become 5.490 cm.
3. Rules of Significant Figures
There are certain significant figures and You need to follow them while doing the calculations. The significant figure calculator calculates the rounded figure of a number.
3.1 Rule of Non-Zero Numbers
You need to consider all the non-zero numbers as significant numbers regardless of their position in the number. You consider them significant and there is always an impact of these numbers in the estimation. The significant digits calculator makes the rounding simple to understand for students.
Example
- Now the repeating non-zero number 2 has an impact on the overall estimation of 5.32222223 m/sec
3.2 Rule of Zero Numbers
When one or more zeros are present between non-zero digits, they are considered significant. They affect the value of the number and the outcome of calculations.
Example
- The zeros coming between the non-zero decimal numbers become significant as they can be observed due to their position in the figure like 5.50005 m/sec
3.3 Rule of Leading Zero
The leading Zeroes coming after the non-zero numbers are considered to be insignificant in the estimation as they have no role in the total estimation or calculations.
Example
- Consider the number 5.5000 m/sec, here all the leading Zeros are insignificant as they have no impact on the overall estimation.
3.4 Rule of Trailing Zero
The trailing Zeros would become significant if it has a decimal after them and these Zeroes are considered significant in the estimation and have an impact on the estimation.
Example 1:
- Consider the number 5.0001 m/sec, now these Zeros would become significant numbers.
Example 2:
- You can't read a long figure like 3.2543331, easily. Therefore, you need to round off the figure to understand.
- The number 3.2543331 is accurate but not easy to understand. You round off the figure and make it 3.25 and make it an easy number to understand for ourselves.
- You can now easily understand and use the rounded number 3.25 in your calculations.
These rules would be consistent when you are calculating the significant figure in the estimations. The significant figures calculator makes the estimation easy for you. These tools can implement these tools on long figures and you can handle and extract the result precisely when dealing with the significant figure in the workplace.
Conclusion
When you are doing the estimation in the lab, you may be dealing with the smallest figure or dealing with large numbers. In both cases, you do need the significant figure to make our estimation more precise and easy to understand. The sig fig calculator makes the task much simpler for you.